While I was reading stuff for yesterday’s post on Egyptian fractions, the name Sierpiński popped up a couple of times – and as his triangle is one of my favourite bits of maths, I thought I’d run with that thread for today’s post.
Polish mathematician Wacław Sierpiński has three fractals named after him: the aforementioned triangle, the carpet and the curve. Making Sierpiński triangles is an end-of-year activity I love doing, as we get to go a bit off-curriculum and talk about fractals – one enthusiastic group once constructed a large triangle from their smaller ones that covered most of the wall in my old classroom, and making bunting from it also looks pretty funky.
Draw an equilateral triangle and mark the midpoints of each side. Connect these midpoints to create another equilateral triangle inside the first one. “Remove” (i.e. shade if you’re colouring it) the smaller triangle you’ve just constructed. You’re then left with three other equilateral triangles – and just repeat the process. It works best on triangular spotty paper as you can just follow the dots to draw the sides of the triangle.
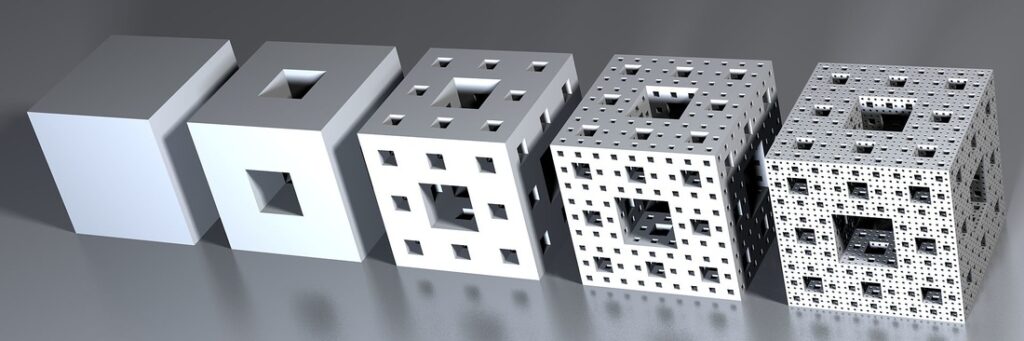
The Sierpiński carpet is essentially made by doing the same thing with a square – but it gets interesting when you move to three dimensions and do the same thing with a cube, creating something called a Menger sponge. If you think of a 3x3x3 cube (like a Rubik’s cube), this amounts to removing the small cube right in the middle, along with every center cube on each face. You then repeat the process with every whole cube that’s left. Infinite repeats give a Menger sponge, which has the strange property of having zero volume but infinite surface area.
Obviously constructing (approximations of) Menger sponges can’t really be done by removing bits from a cube. Check out this page from Dr. Jeannine Mosely, detailing how she and her team made a level 3 sponge from over 66 thousand business cards. There’s also a great video here of the origami club at MIT constructing a sponge for the Mega Menger project run by Queen Mary’s University London. If you can get your hands on old trading cards or pre-cut cardboard, partially constructing a level 2 sponge in the classroom is surprisingly feasible!
The Sierpinski curve is new to me today – I’m kind of seeing it as the flip of the Sierpinski triangle, as rather than removing bits each time, you add on bits instead. Click the link to see it, because although the construction process is simple, it’s impossible to explain. I’m finding the fact that the enclosed area is a neat 5/12 of the original square really fascinating.
(Image credit: “Menger-Schwamm-Reihe” by Niabot – Own work. Licensed under CC BY 3.0 via Wikimedia Commons – https://commons.wikimedia.org/wiki/File:Menger-Schwamm-Reihe.jpg#/media/File:Menger-Schwamm-Reihe.jpg)