Peter Mattock’s session at #mathsconf5 (Concrete Approaches to Abstract Mathematics) was great and gave me a lot of takeaways, so signing up for this one as my first session seemed like a no-brainer. I wasn’t disappointed; this time, Peter looked in depth at fifteen topics that can lead to misleading assumptions by pupils, and challenged us to come up with examples that “broke the mould”.
We started with a little game; Peter asked us to come up with examples we could use to teach particular topics, such as a solvable linear equation or a diagram for teaching parallel line properties. I could sort-of see where this was going, but I decided to embrace the task as it was designed and go for my first idea for each example. We were then awarded points for the predictability of our answers – I scored a whopping 17/20 on my first go with some completely textbook examples. Peter then asked us to improve these examples to come up with a problem set that would score zero. I’ve neatened up (but not censored!) and scanned my notes below, with the original example on the left and improved examples on the right.

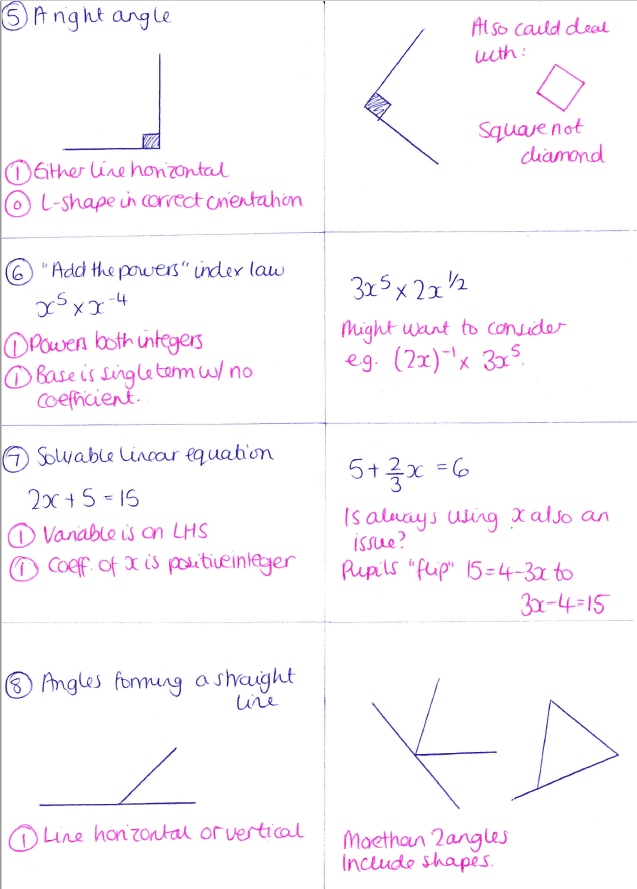
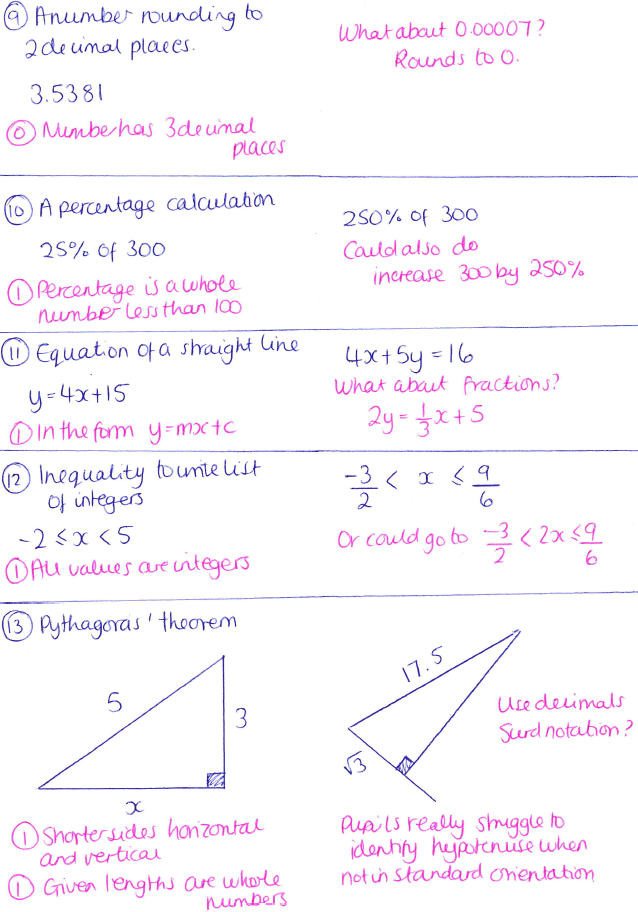
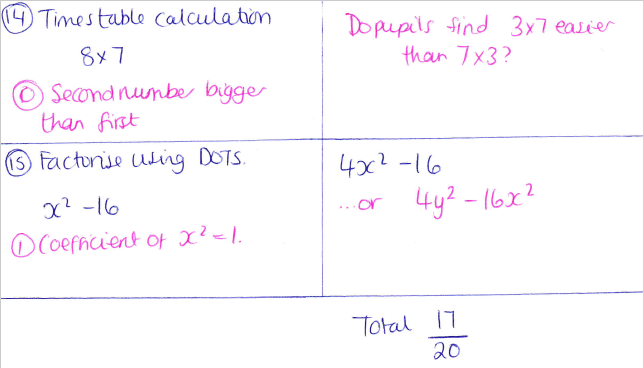
Happily, I’d predicted a few of these as I was working through them. The issues around linear equations in particular caused considerable consternation with my Year 10s a few months ago – they seemed perfectly happy to solve 2x + 5 = 15, but were really confused by 15 = 2x + 5. Many of them decided that they preferred to re-write the equation if it was “backwards” before solving, which worked fine when dealing with all positive terms, but re-writing something like 15 = 5 – 2x caused some of them even more issues, with 2x – 5 = 15 being the most common error.
Personally, I think I sometimes avoid these more complicated problems, particularly when trying to boost confidence with pupils who struggle with mathematics, but this is something I need to work around for myself – pupils need to see more “unfamiliar” examples, particularly now we’re entering the realms of a new, less predictable GCSE.
Peter highlighted the importance of being aware of limitations on our own thinking; while I had predicted some of these assumptions, I hadn’t thought of tilting a simple parallel line diagram or presenting a right angle in a non-standard orientation from the off – this is something I would address later on, often in the context of problem-solving or multi-step questions. However, maybe there is room for this when introducing a new concept, possibly presenting more examples of the right angle L-shape but in non-standard orientations, and specifically pointing out to pupils that these are all right angles.
