Following on from last week’s MathsTLP, I dug out this task on differentiation from first principles – I designed this ages ago for an A Level taster session, but it works equally well to introduce differentiation when it comes up in the A Level syllabus.
So we begin by looking at the gradient of a straight line, to remind ourselves how we calculate as change in y over change in x. I use large delta notation to indicate changes here, as this feeds nicely into use of lower-case delta and eventually, dy/dx notation further on.

Then we look at a simple case of using a line segment to approximate the gradient, and talk about why this isn’t a particularly good approximation – how can we make this better? Well, we make the distance between the two points smaller…


So now we look at decreasing the distance between the two points further. I tend to model this using Desmos, with the point A fixed at (1,1) and a movable point B.
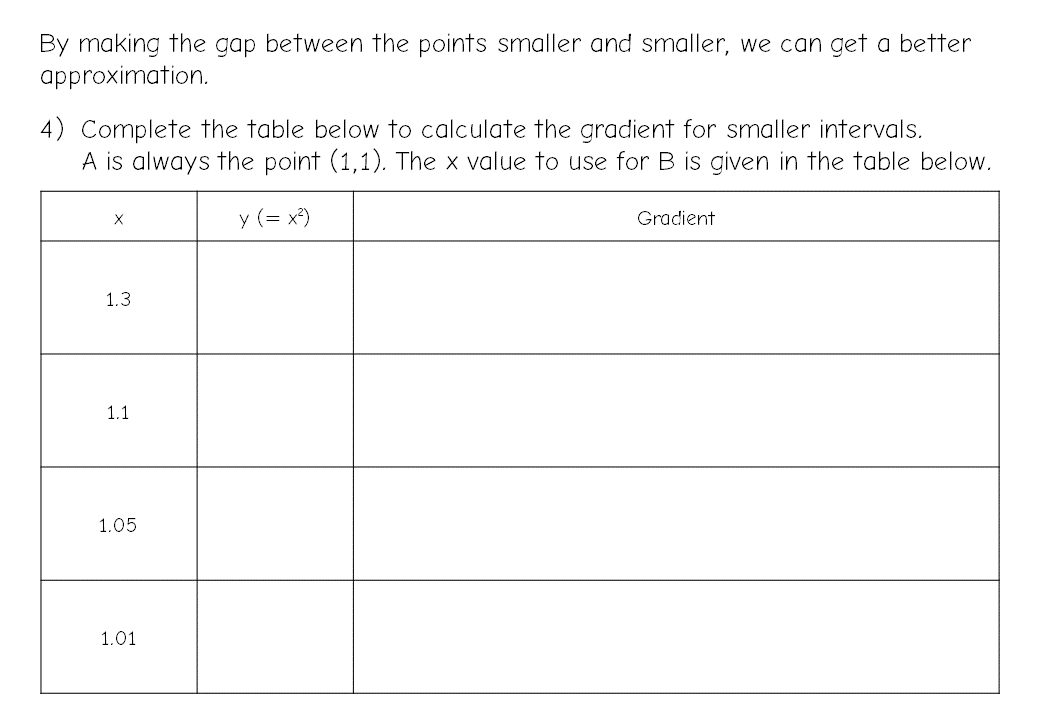
And then we look at what happens for different points. Hopefully students should notice that the values are nearly multiples of 2:

Then we try to develop the general rule. I’d work through this bit slowly with students, modelling each step. The second image shows what I’d roughly be working towards – but I wouldn’t use this typed version in the lesson.


In the original taster session, I didn’t introduce small delta notation or use dy/dx with the students, but it might be appropriate with an A Level group to discuss this notation now. Alternatively this could be left until after the final task:

With A Level students, I’d then go on to discuss correct notation etc and develop the general rule for differentiating polynomials.