These activities were inspired by Adam Boxer’s episode of Mr Barton Maths Podcast – specifically the section in which Adam mentioned teaching speed, distance and time by working with time calculations first. Unfortunately I didn’t record the timestamp, but if anyone fancies listening to the whole thing and letting me know, that would be cool…
Anyway, as I listened I was thinking about ratio tables and how those calculations would look – so here are a few possible activities.
1. What is speed?
Speed is a compound measure, which means it has two (or more) different units. The other biggies at GCSE are density and pressure, and I’m going to be looking at a similar approach to both of these over the next few days.
The idea with this first activity is to emphasise the idea that speed is the distance travelled in a given unit of time (usually minutes or hours). So the first section looks at multiplying up to find whole hour amounts, and the second section emphasises that if you go 60 miles in 60 minutes, you go 30 miles in 30 minutes (and so on).
Whether or not I’d always give learners the first column in the ratio table is up for debate and probably dependent on the group/situation. Entering the information into the first column is the most difficult thing about using ratio tables I reckon! But it’s good to have the discussion about “60 miles per hour means 60 miles in 1 hour” as you enter the “1” into the time row.
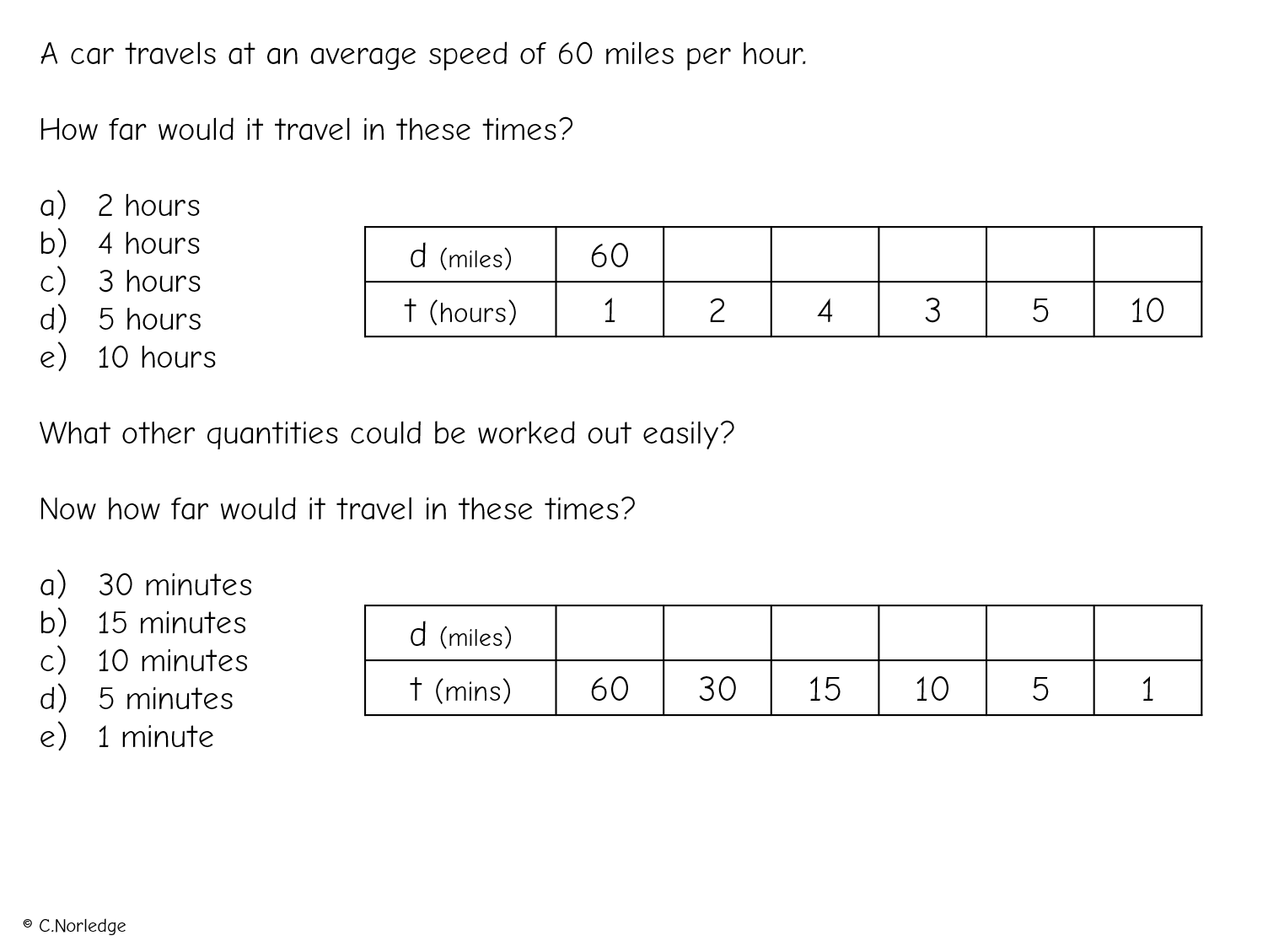
2. Finding times
So now, flipping it round and working out the time taken to travel given distances. The first section is just multiplying up and combining other amounts (so 21 miles from 30 – 9 or similar).
The second section looks at what happens when you start to look at amounts less than one hour. Depending on the group you could tell them to use minutes straight away (as in the ratio table) or just give them a blank space and see if they figure it out for themselves.

3. Hours or minutes?
This is a task specifically designed to show learners that it’s sometimes easier to work the calculation in minutes (and convert to the desired units at the end) than work with fractions of an hour. Of course, some learners might be perfectly happy with 25 miles taking 5/6 of an hour, but I think minutes makes more sense in my head for questions like this one!
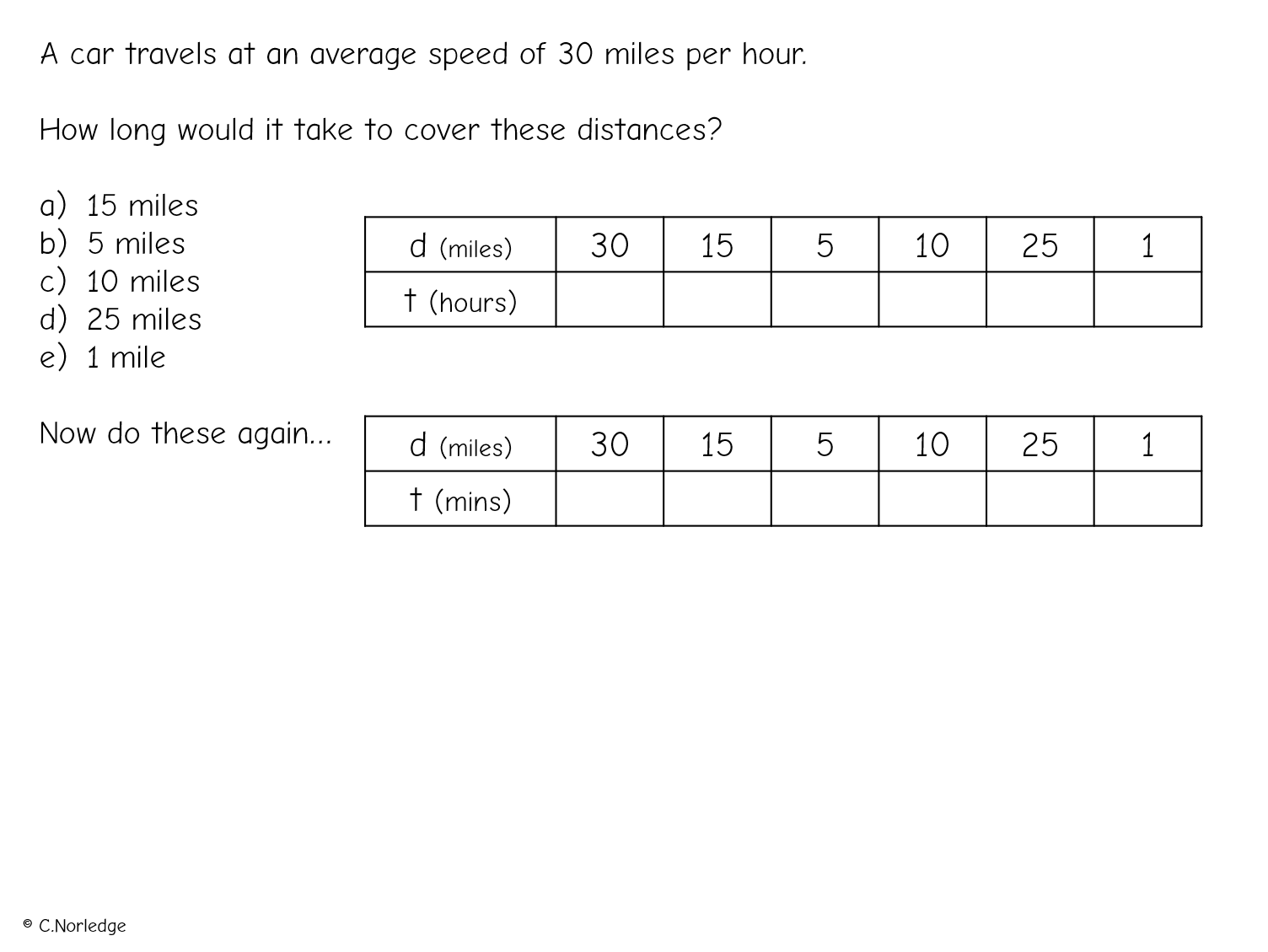
4. Finding speeds
Finally an activity about calculating speed – some multiplying up, some dividing. The time units are blank in c-e as it’s worth having a discussion here about whether to work in hours or minutes.



