1. Crack place value first
This might seem like a no-brainer, but teaching rounding on top of an insecure understanding of place value is a recipe for disaster. Before pupils can get their heads around rounding, particularly to a given number of decimal places, they need to understand why 0.43 is bigger than 0.413, otherwise the whole thing’s a write-off.
2. Work with human number lines
When I teach rounding for the first time, I like to get pupils out at the front with mini-whiteboards to model a number line. For example, I might start with two pupils holding 10 and 20 at either end at the front of the room, then hand intermediate numbers to other pupils and get them to stand in the correct position on the number line. It’s then useful to have a discussion about which end (pupil) they are closest to and how this relates to rounding “to the nearest 10”.
3. Be really careful in discussing what happens with fives
I think it’s important that pupils realise that 15 is exactly in the middle of 10 and 20, and the decision to round up is arbitrary and done by convention. I remember having a 20 minute argument with a group of Year 11s in my training year who were convinced I was teaching upper bounds incorrectly because they’d grabbed onto the idea that “5 always rounds up”, so were seeing it as somehow closer to the next number up, rather than exactly in the middle.
4. Also be really careful about “rounding down”
This is something I’ve only thought about this year when I found several Year 11 pupils “rounding down” by subtracting – i.e. rounding 24.52 (1dp) to 24.4. I’ve now started to say “stays at”, rather than “rounds down”, which seems to be working well.
5. Find a modelling technique that works
Having taught rounding at least seventeen million times, this is how I model it:
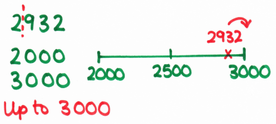
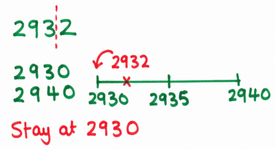
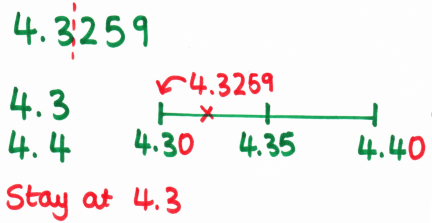
- Mark position of digits you want.
- Explicitly write down which two numbers it is between (e.g. 2932 is between the tens 2930 and 2940).
- Draw a number line; mark the two tens boundaries, the middle and the number we’re rounding.
- Look where it’s closest to; 2932 “stays at” 2930 to the nearest 10, but “rounds up” to 3000 to the nearest 1000.
As pupils get more confident, I gradually drop the number lines, then the boundary values, but keep the dotted line and stay/round up wording.
You can download a number line rounding worksheet here.
6. Reinforce rounding explicitly when teaching topics which naturally require it
When teaching any topics that typically require rounding in exam situations, make sure pupils are getting the rounding right every time. Mix up the rounding required; put some questions to decimal places and some to significant figures.
7. Look for other opportunities when working with whole numbers and decimals
If they’re practising written calculations such as multiplication or division, get them to round their answers as an additional step. Again, mix up the rounding as appropriate for the class currently. Decimal multiplication is particularly good for this.